This work was produced by Curry et al. under the terms of a commissioning contract issued by the Secretary of State for Health and Social Care. This is an Open Access publication distributed under the terms of the Creative Commons Attribution CC BY 4.0 licence, which permits unrestricted use, distribution, reproduction and adaptation in any medium and for any purpose provided that it is properly attributed. See: https://creativecommons.org/licenses/by/4.0/. For attribution the title, original author(s), the publication source – NIHR Journals Library, and the DOI of the publication must be cited.
NCBI Bookshelf. A service of the National Library of Medicine, National Institutes of Health.
Curry N, Davenport R, Thomas H, et al. Early high-dose cryoprecipitate to reduce mortality in adult patients with traumatic haemorrhage: the CRYOSTAT-2 RCT with cost-effectiveness analysis. Southampton (UK): National Institute for Health and Care Research; 2024 Nov. (Health Technology Assessment, No. 28.76.)

Early high-dose cryoprecipitate to reduce mortality in adult patients with traumatic haemorrhage: the CRYOSTAT-2 RCT with cost-effectiveness analysis.
Show detailsAim
The aim of this study was to evaluate the cost-effectiveness of early high-dose cryoprecipitate versus standard-of-care in adult patients with major trauma haemorrhage requiring MHP activation.
Methods
Overview of economic evaluation
We undertook a cost–utility analysis to estimate the within-trial cost-effectiveness of early high-dose cryoprecipitate versus standard-of-care in adult patients with major trauma haemorrhage requiring MHP activation at 28 days following randomisation. The analysis was performed using individual patient-level data from the CRYOSTAT-2 trial. The sample size for the economic analysis was 1581 patients. The outcome measure was the quality-adjusted life-year (QALY), which combines length of life and quality of life, based on the National Institute for Health and Care Excellence (NICE) recommendations.34 Cost-effectiveness was expressed as the incremental cost per QALY gained based on the differences in costs and QALYs between the intervention and standard care arms. The trial was conducted in the UK (25 sites) and the USA (1 site); data from all participants in the study were included, from the UK and the USA. The perspective for the economic analysis was the UK NHS and Personal Social Services. Costs were calculated in 2022–3 Great British pounds and inflated where necessary using the Consumer Price Index.35 The time horizon was 28 days. Extrapolation beyond the end of the trial was not undertaken because the within-trial analysis found no evidence of significant differences in costs or benefits between the intervention and standard care arms; 28 days was long enough to reflect all important differences in costs or outcomes between treatments with early high-dose cryoprecipitate and standard of care. Given the time horizon, discounting was not applied to costs or outcomes.
Resource use and costs
For every patient we calculated costs up to 28 days after randomisation. We included the following items of healthcare resource use that a priori were identified as potentially differing between the two treatment arms:
- length of hospital stay
- number of days in the ICU
- number of days in the high-dependency unit (HDU)
- number of days on an inpatient ward
- use of blood products
- use of RBCs
- use of FFP
- use of cryoprecipitate
- use of platelets
- use of colloids
- use of crystalloids.
Resource use data were collected at 28 days post randomisation using trial CRFs, completed by the trial nurses and patients. The CRF used to collect these data, which are included in Report Supplementary Material 3.36–39
To calculate length of stay in each hospital unit (ICU, HDU, ward), we used the date of admission to that unit and the following date of admission to another unit, or death or discharge. If the patient was not admitted to another unit, or had died or been discharged by the 28th day after randomisation, we assumed that they remained in the latest reported unit until the 28th day. In cases where the patient was known to have been discharged by the 28th day but the discharge date was missing or the duration of stay in a unit was unknown, we assumed that the length of stay in that unit was the mean length of stay observed across all patients. Length of stay after randomisation for both the initial hospitalisation and any re-admissions were included provided that these occurred in the 28 days after randomisation. All length-of-stay calculations were up to 28 days; therefore, any hospital stays beyond this time were not included, even if the patient had not been discharged by 28 days.
Unit costs were obtained from published sources and inflated where appropriate35–38 (Table 9). For length of hospital stay, we multiplied the daily cost by the number of days spent in each type of unit/ward. For blood products, we multiplied the number of units of each product received by the cost per unit.
TABLE 9
Unit costs
Utilities and quality-adjusted life-years
Generic health status was described at 28 days post randomisation using the EQ-5D-5L descriptive system.40 The EQ-5D-5L contains five dimensions: mobility; self-care; usual activities; pain and discomfort; and anxiety and depression. For each dimension of the EQ-5D-5L, there are five levels (no problems, slight problems, moderate problems, severe problems and unable to/extreme problems).33 As patients recruited to the trial were initially critically ill following major trauma, completion of the EQ-5D-5L at randomisation was not possible. Therefore, baseline utility was assumed to be zero for all patients. This assumption was made in previous studies in which baseline utility measurement was not possible.41 EQ-5D-5L health states were converted into utility values using a formula that attaches weights to each level in each dimension based on valuations by UK general population samples;42 this formula was also used for the small proportion of US trial participants. Utility values of one represent full health, values of zero are equivalent to death and negative values represent states worse than death. Patients who died during the 28-day follow-up period were assigned a utility value of zero at 28 days. A utility profile was constructed for every patient, assuming a straight-line relation between their utility values at randomisation (assumed to be zero) and 28 days (measured). QALYs for every patient from baseline to 28 days were calculated as the area under the utility profile. The implications of our assumptions were therefore as follows. For those patients who survived up to 28 days, QALYs up to 28 days were calculated using the EQ-5D-5L scores measured at 28 days, assuming an EQ-5D-5L score of zero at randomisation, and a linear interpolation between randomisation and 28 days. For decedents between randomisation and 28 days, we assumed zero QALYs. We assigned all the EQ-5D-5D data that were collected to the 28-day measurement point, irrespective of the precise time when this was actually measured.
Statistical methods
We addressed missing values of the volume of blood products received and EQ-5D-5L scores at 28 days using multiple imputation for each treatment arm separately.43,44 Patient age, sex, injury type (blunt/penetrating), early receipt of tranexamic acid, ISS and Glasgow Coma Scale were used in the multiple imputation models as additional explanatory variables. We used an iterative Markov chain Monte Carlo procedure based on multivariate normal regression, generating 20 imputed data sets.
We investigated the incremental costs and incremental effectiveness of the intervention using regression analysis based on the participant-level costs and outcomes data. We regressed costs, separately for the costs of hospital stay and cost of blood products, and all cost components combined, against an indicator variable for the intervention arm using generalised linear model regression (gamma family and log link) to account for the skewness of the cost data.45 We regressed QALYs (with and without imputation) against an indicator variable for the intervention arm using ordinary least squares regression. In these regression models, we did not adjust for covariates, and we report the coefficient and marginal effect of the intervention arm (for the analysis of QALYs gained the marginal effect is the coefficient).
The incremental cost per QALY gained was calculated as the incremental cost divided by the QALYs gained; this was computed using the results from the regression analyses as the marginal effect of the coefficient on the indicator variable for the intervention arm in the cost regression model divided by the coefficient on the indicator variable for the intervention arm in the QALY regression model.
We used the ‘multiple imputation nested within bootstrapping’ approach suggested by Brand et al.44 We calculated the mean value for each observation across the 20 imputed data sets and ran the regression analyses described below on this data set. We then reran this analysis 1000 times, using non-parametric bootstrapping, resampling the mean values with replacement.
We repeated the above process investigating cost-effectiveness for subgroups and participants differentiated by sex, age (< 70 years vs. ≥ 70 years) and injury type (blunt vs. penetrating).
We created a scatterplot to display the 1000 bootstrapped replications using the incremental QALYs with imputation. A cost-effectiveness acceptability curve showing the probability that early cryoprecipitate would be cost-effective compared with standard care at a range of values for the maximum willingness to pay for a QALY was generated based on the proportion of these 1000 bootstrap replications with positive incremental net monetary benefits (calculated as the mean incremental QALYs per patient with early cryoprecipitate multiplied by the maximum willingness to pay for a QALY minus the mean incremental cost per patient with early cryoprecipitate).
Results
Resource use and costs
In our sample, the overall mean length of stay in the ICU across all patients was 6.5 days [standard deviation (SD) 8.5 days] and the median was 3 days (IQR 0–10 days) (Table 10). The overall mean length of stay in the HDU was 1.8 days (SD 3.5 days) and the median was 0 days (IQR 0–3 days). The mean length of stay on the ward overall was 5.8 days (SD 7.0 days) and the median was 3 days (IQR 0–10 days). The mean and median total lengths of stay overall across all patients were 14.1 days (SD 11.0 days) and 12 days (IQR 3–28 days), respectively. All length of hospital stay values were similar in the intervention and standard care arms.
TABLE 10
Descriptive statistics for resource use measures
In terms of the volume of blood products received, the mean and median number of RBC units overall across all patients were 6.5 (SD 7.7) and 4 (IQR 2–8), respectively. The mean and median number of FFP units overall were 5.4 (SD 6.9) and 4 (IQR 2–7), respectively. For cryoprecipitate these values were 2.0 (SD 2.5) and 3 (IQR 0–3), respectively. For platelets they were 0.8 (SD 1.5) and 0 (IQR 0–1), respectively. For crystalloids the mean and median volumes in millilitres overall were 2108 (SD 2171) and 1506 (IQR 100–3100), respectively. For colloids they were 99 (SD 521) and 0 (IQR 0–0), respectively. All blood product values were similar for the intervention and standard care arms, with the exception of cryoprecipitate, where the mean and median values were 3.1 (SD 2.4) and 3 (IQR 3–3) in the intervention arm and 1.0 (SD 2.0) and 0 (IQR 0–2) in the standard care arm.
The mean total cost per patient (including the costs of length of hospital stay and blood products) was £19,477 (SD £16,207) in the intervention arm and £19,236 (SD £16,681) in the standard care arm (Table 11). In both arms, approximately 90% of the total cost was accounted for by the cost of hospital stay, and 10% by the cost of blood products. ICU costs accounted for most of the cost of hospital stay, accounting for approximately 70% of the cost of hospital stay and 62% of the total cost.
TABLE 11
Descriptive statistics for costs
Utilities and quality-adjusted life-years
Mean EQ-5D-5L utility scores at 28 days overall across all patients were 0.294 (SD 0.339) (Table 12). Mean QALYs overall were 0.011 (SD 0.013) with no imputation and 0.012 (SD 0.013) with imputation. Both mean EQ-5D-5L utility scores at 28 days and mean QALYs were similar in the intervention and standard care arms.
TABLE 12
EuroQol-5 Dimensions, five-level version utility scores and QALYs
Incremental cost–utility analysis
The results of the regression analyses for costs across the whole sample indicate that there were significant differences in the cost of blood products between the intervention and standard care arms (costs were £519 higher in the intervention arm; p < 0.0001; Table 13). The differences between the costs associated with the length of hospital stay and the total costs were not statistically significant in either of the two arms (both p > 0.7). The results of the regression analyses for QALYs (without and with imputation) indicate that the between-arm differences in QALYs were small (both QALYs gained < 0.0002) and not statistically significant (both p > 0.7).
TABLE 13
Results of regression analyses for costs and QALYs
The incremental cost per QALY gained for intervention versus standard care is £1,121,509 based on the QALYs gained calculated without imputation and £1,348,181 based on the QALYs gained calculated with imputation (numbers may not be directly calculated from the values in Table 13 due to rounding). The high, unattractive incremental cost-effectiveness ratios associated with early cryoprecipitate compared with standard MHP are due to the higher cost of blood products that is not offset by lower costs elsewhere, and the negligible QALY gains/losses.
In terms of the subgroup analyses (Tables 14–16), the regression analyses were largely qualitatively the same as for the whole sample: the cost of blood products was significantly higher for the intervention arm; for all other cost variables and the QALY variables there were no significant differences between early cryoprecipitate and standard MHP, and the QALYs gained were negligible in magnitude, making the interpretation of the incremental cost per QALY gained difficult.
TABLE 14
Results of regression analyses for costs and QALYs: subgroup analysis by sex
TABLE 16
Results of regression analyses for costs and QALYs: subgroup analysis by age group
TABLE 15
Results of regression analyses for costs and QALYs: subgroup analysis by injury type
The scatterplot showing the bootstrap replications is shown in Figure 9. The cost-effectiveness acceptability curve is shown in Figure 10. This shows that the probability that early cryoprecipitate is cost-effective versus standard MHP is just under 0.4 across a range of plausible values of the cost-effectiveness threshold.
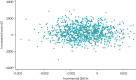
FIGURE 9
Scatterplot showing 1000 bootstrap replications of the incremental costs and QALYs associated with early cryoprecipitate vs. standard MHP.
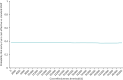
FIGURE 10
Cost-effectiveness acceptability curve showing the probability that early cryoprecipitate is cost-effective vs. standard MHP for a range of cost-effectiveness thresholds.
Summary
Our economic analysis to evaluate the cost-effectiveness of using early high-dose cryoprecipitate versus standard of care in adult patients with major trauma haemorrhage requiring MHP activation showed that there are no differences in terms of costs and outcomes. The findings mean that there is no reason to prefer early high-dose cryoprecipitate to standard of care based on cost or value-for-money grounds.
- Economic evaluation - Early high-dose cryoprecipitate to reduce mortality in adu...Economic evaluation - Early high-dose cryoprecipitate to reduce mortality in adult patients with traumatic haemorrhage: the CRYOSTAT-2 RCT with cost-effectiveness analysis
Your browsing activity is empty.
Activity recording is turned off.
See more...